Inverse Fisher Transform COMBO STO+RSI+CCIv2 by KIVANÇ fr3762

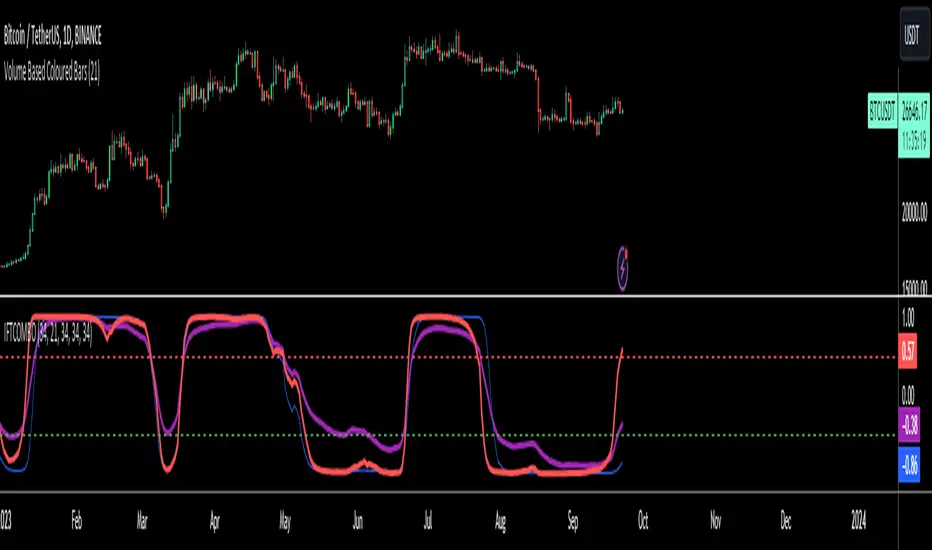
A combined 3in1 version of pre shared INVERSE FISHER TRANSFORM indicators on RSI , on STOCHASTIC and on CCIv2 to provide space for 2 more indicators for users...



About John EHLERS:


From California, USA, John is a veteran trader. With 35 years trading experience he has seen it all. John has an engineering background that led to his technical approach to trading ignoring fundamental analysis (with one important exception).
John strongly believes in cycles. He’d rather exit a trade when the cycle ends or a new one starts. He uses the MESA principle to make predictions about cycles in the market and trades one hundred percent automatically.
In the show John reveals:
• What is more appropriate than trading individual stocks
• The one thing he relies upon in his approach to the market
• The detail surrounding his unique trading style
• What important thing underpins the market and gives every trader an edge


About INVERSE FISHER TRANSFORM:

The purpose of technical indicators is to help with your timing decisions to buy or
sell. Hopefully, the signals are clear and unequivocal. However, more often than
not your decision to pull the trigger is accompanied by crossing your fingers.
Even if you have placed only a few trades you know the drill.
In this article I will show you a way to make your oscillator-type indicators make
clear black-or-white indication of the time to buy or sell. I will do this by using the
Inverse Fisher Transform to alter the Probability Distribution Function ( PDF ) of
your indicators. In the past12 I have noted that the PDF of price and indicators do
not have a Gaussian, or Normal, probability distribution. A Gaussian PDF is the
familiar bell-shaped curve where the long “tails” mean that wide deviations from
the mean occur with relatively low probability. The Fisher Transform can be
applied to almost any normalized data set to make the resulting PDF nearly
Gaussian, with the result that the turning points are sharply peaked and easy to
identify. The Fisher Transform is defined by the equation
1)
Whereas the Fisher Transform is expansive, the Inverse Fisher Transform is
compressive. The Inverse Fisher Transform is found by solving equation 1 for x
in terms of y. The Inverse Fisher Transform is:
2)
The transfer response of the Inverse Fisher Transform is shown in Figure 1. If
the input falls between –0.5 and +0.5, the output is nearly the same as the input.
For larger absolute values (say, larger than 2), the output is compressed to be no
larger than unity . The result of using the Inverse Fisher Transform is that the
output has a very high probability of being either +1 or –1. This bipolar
probability distribution makes the Inverse Fisher Transform ideal for generating
an indicator that provides clear buy and sell signals.

Creator: John EHLERS

릴리즈 노트:

SHOW/REMOVE button added to settings

Inverse Fisher transform on CCIv2 will defaultly appear on the screen, users can add orr remove INVERSE FISHER TRANSFORM on RSI and INVERSE FISHER TRANSFORM on STOCHASTIC by clicking the box on the settings menu

릴리즈 노트:

AVERAGE LINE added to the indicator (arithmetic mean of all 3 inverse transformed indicators)
AVERAGE LINE = (inverse cciv2+ inverse rsi+ inverse stochastic)/3

users can make the AVERAGE LINE appear, in purple if the AVERAGE box is selected in the settings.

릴리즈 노트:

Added option to plot the Inverse fisher transform on MFI and also users can plot the average of 4 indicators if they prefer to select the AVERAGE option

릴리즈 노트:

graphical update

릴리즈 노트:

Updated to v5 and added multiple Time frame Feature.

릴리즈 노트:

minor update